PHYSICS PROJECT REPORT ON LEVER

A lever (/ˈliːvər/ or US: /ˈlɛvər/) is a simple machine consisting of a beam or rigid rod pivoted at a fixed hinge, or fulcrum. A lever is a rigid body capable of rotating on a point on itself. On the basis of the location of fulcrum, load and effort, the lever is divided into three types. It is one of the six simple machines identified by Renaissance scientists. A lever amplifies an input force to provide a greater output force, which is said to provide leverage. The ratio of the output force to the input force is the mechanical advantage of the lever.

Etymology[edit]

The word “lever” entered English about 1300 from Old French, in which the word was levier. This sprang from the stem of the verb lever, meaning “to raise”. The verb, in turn, goes back to the Latin levare, itself from the adjective levis, meaning “light” (as in “not heavy”). The word’s primary origin is the Proto-Indo-European (PIE) stem legwh-, meaning “light”, “easy” or “nimble”, among other things. The PIE stem also gave rise to the English word “light”.^[1]

Early use[edit]

The earliest remaining writings regarding levers date from the 3rd century BCE and were provided by Archimedes. ‘Give me a place to stand, and I shall move the Earth with it’ is a remark of Archimedes who formally stated the correct mathematical principle of levers (quoted by Pappus of Alexandria).

It is assumed^{[by whom?]} that in ancient Egypt, constructors used the lever to move and uplift obelisks weighing more than 100 tons.

Force and levers[edit]

A lever is a beam connected to ground by a hinge, or pivot, called a fulcrum. The ideal lever does not dissipate or store energy, which means there is no friction in the hinge or bending in the beam. In this case, the power into the lever equals the power out, and the ratio of output to input force is given by the ratio of the distances from the fulcrum to the points of application of these forces. This is known as the law of the lever.

The mechanical advantage of a lever can be determined by considering the balance of moments or torque, T, about the fulcrum.

{\displaystyle T_{1}=F_{1}a,\!} {\displaystyle T_{2}=F_{2}b\!}

where F₁ is the input force to the lever and F₂ is the output force. The distances a and b are the perpendicular distances between the forces and the fulcrum.

Since the moments of torque must be balanced, {\displaystyle T_{1}=T_{2}\!} . So, {\displaystyle F_{1}a=F_{2}b\!}.

The mechanical advantage of the lever is the ratio of output force to input force,

{\displaystyle MA={\frac {F_{2}}{F_{1}}}={\frac {a}{b}}.\!}

This relationship shows that the mechanical advantage can be computed from ratio of the distances from the fulcrum to where the input and output forces are applied to the lever, assuming no losses due to friction, flexibility or wear. This remains true even though the horizontal distance (perpendicular to the pull of gravity) of both a and b change (diminish) as the lever changes to any position away from the horizontal.

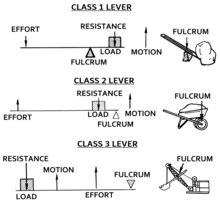
Classes of levers[edit]

Levers are classified by the relative positions of the fulcrum, effort and resistance (or load). It is common to call the input force the effort and the output force the load or the resistance. This allows the identification of three classes of levers by the relative locations of the fulcrum, the resistance and the effort:^[2]

• Class 1: Fulcrum in the middle: the effort is applied on one side of the fulcrum and the resistance (or load) on the other side, for example, a seesaw, a crowbar or a pair of scissors. Mechanical advantage may be greater than, less than, or equal to 1.
• Class 2: Resistance (or load) in the middle: the effort is applied on one side of the resistance and the fulcrum is located on the other side, for example, a wheelbarrow, a nutcracker, a bottle opener or the brake pedal of a car. Load arm is smaller than the effort arm. Mechanical advantage is always greater than 1. It is also called force multiplier lever.
• Class 3: Effort in the middle: the resistance (or load) is on one side of the effort and the fulcrum is located on the other side, for example, a pair of tweezers or the human mandible. The effort arm is smaller than the load arm. Mechanical advantage is always less than 1. It is also called speed multiplier lever.

These cases are described by the mnemonic fre 123 where the fulcrum is in the middle for the 1st class lever, the resistance is in the middle for the 2nd class lever, and the effort is in the middle for the 3rd class lever.

Law of the lever[edit]

The lever is a movable bar that pivots on a fulcrum attached to a fixed point. The lever operates by applying forces at different distances from the fulcrum, or a pivot.

Assuming the lever does not dissipate or store energy, the power into the lever must equal the power out of the lever. As the lever rotates around the fulcrum, points farther from this pivot move faster than points closer to the pivot. Therefore, a force applied to a point farther from the pivot must be less than the force located at a point closer in, because power is the product of force and velocity.^[3]

If a and b are distances from the fulcrum to points A and B and the force F_A applied to A is the input and the force F_B applied at B is the output, the ratio of the velocities of points Aand B is given by a/b, so we have the ratio of the output force to the input force, or mechanical advantage, is given by

{\displaystyle MA={\frac {F_{B}}{F_{A}}}={\frac {a}{b}}.}

This is the law of the lever, which was proven by Archimedes using geometric reasoning.^[4] It shows that if the distance a from the fulcrum to where the input force is applied (point A) is greater than the distance b from fulcrum to where the output force is applied (point B), then the lever amplifies the input force. On the other hand, if the distance a from the fulcrum to the input force is less than the distance b from the fulcrum to the output force, then the lever reduces the input force.

The use of velocity in the static analysis of a lever is an application of the principle of virtual work.

Virtual work and the law of the lever[edit]

A lever is modeled as a rigid bar connected to a ground frame by a hinged joint called a fulcrum. The lever is operated by applying an input force F_A at a point A located by the coordinate vector r_A on the bar. The lever then exerts an output force F_B at the point B located by r_B. The rotation of the lever about the fulcrum P is defined by the rotation angle θ in radians.

Let the coordinate vector of the point P that defines the fulcrum be r_P, and introduce the lengths

{\displaystyle a=|\mathbf {r} _{A}-\mathbf {r} _{P}|,\quad b=|\mathbf {r} _{B}-\mathbf {r} _{P}|,}

which are the distances from the fulcrum to the input point A and to the output point B, respectively.

Now introduce the unit vectors e_A and e_B from the fulcrum to the point A and B, so

{\displaystyle \mathbf {r} _{A}-\mathbf {r} _{P}=a\mathbf {e} _{A},\quad \mathbf {r} _{B}-\mathbf {r} _{P}=b\mathbf {e} _{B}.}

The velocity of the points A and B are obtained as

{\displaystyle \mathbf {v} _{A}={\dot {\theta }}a\mathbf {e} _{A}^{\perp },\quad \mathbf {v} _{B}={\dot {\theta }}b\mathbf {e} _{B}^{\perp },}

where e_A^⊥ and e_B^⊥ are unit vectors perpendicular to e_A and e_B, respectively.

The angle θ is the generalized coordinate that defines the configuration of the lever, and the generalized force associated with this coordinate is given by

{\displaystyle F_{\theta }=\mathbf {F} _{A}\cdot {\frac {\partial \mathbf {v} _{A}}{\partial {\dot {\theta }}}}-\mathbf {F} _{B}\cdot {\frac {\partial \mathbf {v} _{B}}{\partial {\dot {\theta }}}}=a(\mathbf {F} _{A}\cdot \mathbf {e} _{A}^{\perp })-b(\mathbf {F} _{B}\cdot \mathbf {e} _{B}^{\perp })=aF_{A}-bF_{B},}

where F_A and F_B are components of the forces that are perpendicular to the radial segments PA and PB. The principle of virtual work states that at equilibrium the generalized force is zero, that is

{\displaystyle F_{\theta }=aF_{A}-bF_{B}=0.\,\!}

Thus, the ratio of the output force F_B to the input force F_A is obtained as

{\displaystyle MA={\frac {F_{B}}{F_{A}}}={\frac {a}{b}},}

which is the mechanical advantage of the lever.

This equation shows that if the distance a from the fulcrum to the point A where the input force is applied is greater than the distance b from fulcrum to the point B where the output force is applied, then the lever amplifies the input force. If the opposite is true that the distance from the fulcrum to the input point A is less than from the fulcrum to the output point B, then the lever reduces the magnitude of the input force.

LOOKING FOR A WORKING MODEL FOR SCHOOL OR COLLEGE?

12th class is a no risk class. It is the class which decide your future. Whether you are going to work in MNC or going to work with usual private firms, it all depends here. To ensure the good marks to students we always stands behind them with all practical and theoretical support on Physics investigatory project. These Physics investigatory projects are usually have to be made by all students with physics and to be shown in boards practical. We at Gurukul Technicals Institute provide every student the theory behind the projects and give all possible required material to make the project work successfully. We duly provide equipped lab with latest lab materials. Conductors and Semiconductors are provided with Circuit diagram so that student can make project easily. Students make projects such as Full wave rectifier, Half wave rectifier, Fire alarm, rain alarm, Electromagnetic Induction, Logic gates(AND gate, OR gate, NOT gate, XAND gate, XNOR gate), Transistor as switch, Transistor as amplifier, Charging and Discharging of Capacitors, Series and Parallel Resistance etc etc. Students also get help on making these above given projects to made on cardboard or on breadboard as instructed by teacher. Students are also welcome to take help on report on given physics investigatory projects. After reading this website you can also send this information to your friend or relative studying in 12th class so it will make useful to him/her.

Get Readymade Project anywhere in India on just a Click away! That too with Report!

Prompt Delivery!  COD Available!  All over India Delivery! Order Now!!

CLICK HERE TO VIEW ALL 12th CLASS INVESTIGATORY PROJECT WITH REPORT LISTED ON AMAZON.IN WEBSITE

 

CLICK HERE TO VIEW MINOR,MAJOR AND ALL OTHER INVESTIGATORY PROJECTS ON FLIPKART.COM WEBSITE

Physics Projects with Reports:

1. TO CONSTRUCT A CIRCUIT FOR TOUCH ALARM.
2. EDDY CURRENT {WITHOUT MODEL }
3. PHOTOCONDUCTIVE CELL
4. MUTUAL INDUCTION
5. TO STUDY NPN-TRANSISTOR AMPLIFIER
6. TO CONSTRUCT A ELECTRIC MOTORS (D.C.MOTOR)
7. TO STUDY HOW A TRANSISTOR AMPLIFIER WORK ‘PNP’ AMPLIFIER TRANSISTOR.
8. HOUSEHOLD CIRCUITS
9. AC TO DC CONVERTER (FULL WAVE RECTIFIER)
10. AC TO DC CONVERTER (HALF WAVE RECTIFIER)
11. TO CONSTRUCT A CIRCUIT OF FENCE WIRE BURGLAR ALARM
12. TO SHOW THE PRINCIPLE OF FARADAY’S AND A.C. GENERATOR.
13. THERMOCOUPLE
14. TO STUDY FARADAY’S LAWS-TO FIND THE CHARGE ON AN ELECTRON
15. FARADAY’S LAW’S OF ELECTROLYSIS
16. TO STUDY A POSITIVE FEED BACK CIRCUIT OF AN AUDIO OSCILLATOR (LC OSCILLATOR)
17. HOW DOES AN ELECTRIC GENERATOR WORK
18. TO SHOW THAT A SOLENOID CARRYING AN ELECTRIC CURRENT PRODUCES A MAGNETIC FIELD SIMILAR TO THAT PRODUCED BY A BAR MAGNET.
19. ELECTROCHEMICAL CELL (PRIMARY CELL)
20. BOOLAN LOGIC GATE
21. TO STUDY THE CHARGE AND DISCHARGING OF CAPACITOR IN SERIES
22. WORKING OF POTENTIOMETER
23. KIRCHOFF’S LAW
24. TO DEMONSTRATE THE WORKING OF AN ELECTROLYTIC CAPACITOR BY MEANS OF ITS CHARGING AND DISCHARGING WITH THE HELP OF AN AUDIO OSCILLATOR AND TO STUDY AND COMPARE THE TWO CAPACITORS UNDER SERIES AND PARALLEL COMBINATION.(Z)
25. RADIOACTIVITY AND NUCLEAR REACTIONS.
26. RADIOISOTOPE THERMOELECTRIC GENERATOR.
27. TO CONSTRUCT A CIRCUIT OF SOUND OPERATED SWITCH.
28. TO CONSTRUCT A CIRCUIT OF TIME OPERATED SWITCH.
29. TO CONSTRUCT A CIRCUIT OF SOUND AMPLIFIER.
30. TO CONSTRUCT A CIRCUIT OF A FIRE ALARM.
31. TO CONSTRUCT A CIRCUIT OF CLAP SWITCH.
32. TO CONSTRUCT A CIRCUIT OF TRANSISTOR SWITCH
33. TO FIND OUT OPTICAL ACTIVITY ARISES WHEN THE POLARIZATION AXIS OF LIGHT IS ROTATED AS IT PASSES THROUGH A SUBSTANCE
34. PUSH-PULL AMPLIFIER
35. HARTLEY OSCILLATORS
36. PUSH PULL AMPLIFIER
37. REPORT ON MAGNETIC FIELDS, MAGNETIC FORCES, AND ELECTROMAGNETIC INDUCTION LAWS OF REFRACTION (Z).
38. RAY OPTICS-TO FIND REFRACTIVE INDEX OF THE MATERIAL OF THE PRISM BY TOTAL INTERNAL REFRACTION (Z).
39. TO DEMONSTRATE THE WORKING OF AN ELECTROLYTIC CAPACITOR BY MEANS OF ITS CHARGING AND DISCHARGING WITH THE HELP OF AN AUDIO OSCILLATOR AND TO STUDY AND COMPARE THE TWO CAPACITORS UNDER SERIES AND PARALLEL COMBINATIONS.
40. TO STUDY THE EFFECT OF THE DIAMETER AND THE NUMBER OF TURNS OF THE SPRING ON ITS STRENGTH HAS BEEN INVESTIGATION IN THIS STUDY.(Z)
41. TO FIND OUT THE THERMAL COEFFICIENT OF RESISTANCE FOR A DIVAN SET OF WIRES AND THUS SUGGEST THE WIRE IN WHICH ENERGY LOSS DUE TO HEAT GENERATION IS MINIMUN.(Z)
42. TO SEE THAT WATER CONDUCTS ELECTRICITY BETTER WHEN IMPURITIES ARE ADDED IT. (Z)
43. TO MAKE NOR GATE WITH THE COMBINATION OF TWO GATES. (Z)
44. PASCAL’S LAW AND ITS APPLICATIONS
45. BRIDGE RECTIFIER- A CIRCUIT USING FOUR DIODES TO PROVIDE FULL WAVE RECTIFICATION.CONVERTS AN AC VOLTAGE TO A PULSATING DC VOLTAGE.
46. TO CONSTRUCT A CIRCUIT OF QUIZ BUZZER.
47. TO CONSTRUCT A CIRCUIT OF LASER SECURITY SYSTEM.
48. TO CONSTRUCT A CIRCUIT OF RAIN ALARM.
49. TO CONSTRUCT A CIRCUIT OF WATER LEVEL INDICATOR.
50. THE EFFECT OF TEMPERATURE ON DISPOSABLE AND NON DISPOSABLE BATTERIES
51. TO INVESTIGATE THE EFFECT OF THE FOLLOWING FACTORS ON THE INTERNAL RESISTANCE OF A LACLANCHE CELL.
52. TO CONSTRUCT A CIRCUIT OF OPTICAL SWITCHING.
53. TO CONSTRUCT A CIRCUIT OFCAPACITOR CHARGE OSCILATOR
54. TO CONSTRUCT A CIRCUIT OFCAPACITOR STORAGE LED
55. TO CONSTRUCT A CIRCUIT OF TWO TRANSISTOR OSCILLATOR
56. EXPERIMENTS IN ELECTROCHEMISTRY
57. TO SHOW THE UNIDIRECTION ACTION OF DIODE
58. OPTICAL FIBER COMMUNICATION
59. TO CONSTRUCT A CIRCUIT OF ELECTRONIC EYE

NOT IN THE LIST? WHATSAPP ON  9711699511 SO WE CAN SPECIALLY MADE IT FOR YOU. EITHER ON MULTIPURPOSE PCB OR BREAD BOARD.