Projectile motion is a form of motion experienced by an object or particle (a projectile) that is thrown near the Earth’s surface and moves along a curved path under the action of gravity only (in particular, the effects of air resistance are assumed to be negligible). This curved path was shown by Galileo to be a parabola. The study of such motions is called ballistics, and such a trajectory is a ballistic trajectory. The only force of significance that acts on the object is gravity, which acts downward, thus imparting to the object a downward acceleration. Because of the object’s inertia, no external horizontal force is needed to maintain the horizontal velocity component of the object. Taking other forces into account, such as friction from aerodynamic drag or internal propulsion such as in a rocket, requires additional analysis. A ballistic missile is a missile only guided during the relatively brief initial powered phase of flight, and whose subsequent course is governed by the laws of classical mechanics.

Ballistics (gr. βάλλειν (‘ba’llein’), “to throw”) is the science of mechanics that deals with the flight, behavior, and effects of projectiles, especially bullets, unguided bombs, rockets, or the like; the science or art of designing and accelerating projectiles so as to achieve a desired performance.

The elementary equations of ballistics neglect nearly every factor except for initial velocity and an assumed constant gravitational acceleration. Practical solutions of a ballistics problem often require considerations of air resistance, cross winds, target motion, varying acceleration due to gravity, and in such problems as launching a rocket from one point on the Earth to another, the rotation of the Earth. Detailed mathematical solutions of practical problems typically do not have closed-form solutions, and therefore require numerical methods to address.

The initial velocity

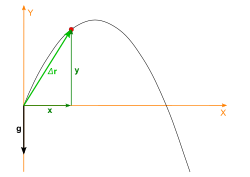
Let the projectile be launched with an initial velocity {\displaystyle \mathbf {v} (0)\equiv \mathbf {v} _{0}}, which can be expressed as the sum of horizontal and vertical components as follows:

{\displaystyle \mathbf {v} _{0}=v_{0x}\mathbf {i} +v_{0y}\mathbf {j} }.

The components {\displaystyle v_{0x}} and {\displaystyle v_{0y}} can be found if the initial launch angle, {\displaystyle \theta }, is known:

{\displaystyle v_{0x}=v_{0}\cos \theta },

{\displaystyle v_{0y}=v_{0}\sin \theta }.

Kinematic quantities of projectile motion

In projectile motion, the horizontal motion and the vertical motion are independent of each other; that is, neither motion affects the other. This is the principle of compound motionestablished by Galileo in 1638.

Acceleration

Since there is only acceleration in the vertical direction, the velocity in the horizontal direction is constant, being equal to {\displaystyle \mathbf {v} _{0}\cos \theta }. The vertical motion of the projectile is the motion of a particle during its free fall. Here the acceleration is constant, being equal to g.The components of the acceleration are-

{\displaystyle a_{x}=0},

{\displaystyle a_{y}=-g}.

Velocity

The horizontal component of the velocity of the object remains unchanged throughout the motion. The vertical component of the changes linearly,because the acceleration due to gravity is constant. The accelerations in the x and y directions can be integrated to solve for the components of velocity at any time t, as follows:

{\displaystyle v_{x}=v_{0}\cos(\theta )},

{\displaystyle v_{y}=v_{0}\sin(\theta )-gt}.

The magnitude of the velocity (under the Pythagorean theorem, also known as the triangle law):

{\displaystyle v={\sqrt {v_{x}^{2}+v_{y}^{2}\ }}}.

Displacement

At any time {\displaystyle t}, the projectile’s horizontal and vertical displacement are:

{\displaystyle x=v_{0}t\cos(\theta )},

{\displaystyle y=v_{0}t\sin(\theta )-{\frac {1}{2}}gt^{2}}.

The magnitude of the displacement is:

{\displaystyle \Delta r={\sqrt {x^{2}+y^{2}\ }}}.

Consider the equations,

{\displaystyle x=v_{0}t\cos(\theta ),y=v_{0}t\sin(\theta )-{\frac {1}{2}}gt^{2}}.

If t is eliminated between these two equations the following equation is obtained:

{\displaystyle y=\tan(\theta )\cdot x-{\frac {g}{2v_{0}^{2}\cos ^{2}\theta }}\cdot x^{2}}.

Since g, θ, and v₀ are constants, the above equation is of the form

{\displaystyle y=ax+bx^{2}},

in which a and b are constants. This is the equation of a parabola, so the path is parabolic. The axis of the parabola is vertical.

If the projectile’s position (x,y) and launch angle (θ or α) are known, the initial velocity can be found solving for v₀ in the aforementioned parabolic equation:

{\displaystyle v_{0}={\sqrt {{x^{2}g} \over {x\sin 2\theta -2y\cos ^{2}\theta }}}}.

Time of flight or total time of the whole journey

The total time t for which the projectile remains in the air is called the time of flight.

{\displaystyle y=v_{0}t\sin(\theta )-{\frac {1}{2}}gt^{2}}

After the flight, the projectile returns to the horizontal axis (x-axis), so {\displaystyle y=0}.

{\displaystyle 0=v_{0}t\sin(\theta )-{\frac {1}{2}}gt^{2}}

{\displaystyle v_{0}t\sin(\theta )={\frac {1}{2}}gt^{2}}

{\displaystyle v_{0}\sin(\theta )={\frac {1}{2}}gt}

{\displaystyle t={\frac {2v_{0}\sin(\theta )}{g}}}

Note that we have neglected air resistance on the projectile.

If the starting point is at height y₀ with respect to the point of impact, the time of flight is:

{\displaystyle t={\frac {d}{v\cos \theta }}={\frac {v\sin \theta +{\sqrt {(v\sin \theta )^{2}+2gy_{0}}}}{g}}}

As above, this expression can be reduced to

{\displaystyle t={\frac {v\sin {\theta }+{\sqrt {(v\sin {\theta })^{2}}}}{g}}={\frac {v\sin {\theta }+v\sin {\theta }}{g}}={\frac {2v\sin {\theta }}{g}}={\frac {2v\sin {(45)}}{g}}={\frac {2v{\frac {\sqrt {2}}{2}}}{g}}={\frac {{\sqrt {2}}v}{g}}}

if θ is 45° and y₀ is 0.

Maximum height of projectile[edit]

The greatest height that the object will reach is known as the peak of the object’s motion. The increase in height will last until {\displaystyle v_{y}=0}, that is,

{\displaystyle 0=v_{0}\sin(\theta )-gt_{h}}.

Time to reach the maximum height(h):

{\displaystyle t_{h}={\frac {v_{0}\sin(\theta )}{g}}}.

From the vertical displacement of the maximum height of projectile:

{\displaystyle h=v_{0}t_{h}\sin(\theta )-{\frac {1}{2}}gt_{h}^{2}}

{\displaystyle h={\frac {v_{0}^{2}\sin ^{2}(\theta )}{2g}}} .

Relation between horizontal range and maximum height

The relation between the range R on the horizontal plane and the maximum height h reached at {\displaystyle {\frac {t_{d}}{2}}} is:

{\displaystyle h={\frac {R\tan \theta }{4}}}

Proof

{\displaystyle h={\frac {v_{0}^{2}\sin ^{2}\theta }{2g}}}

{\displaystyle R={\frac {v_{0}^{2}\sin 2\theta }{g}}}

{\displaystyle {\frac {h}{R}}={\frac {v_{0}^{2}\sin ^{2}\theta }{2g}}} × {\displaystyle {\frac {g}{v_{0}^{2}\sin 2\theta }}}

{\displaystyle {\frac {h}{R}}={\frac {\sin ^{2}\theta }{4\sin \theta \cos \theta }}}

{\displaystyle h={\frac {R\tan \theta }{4}}}.

Maximum distance of projectile

The range and the maximum height of the projectile does not depend upon its mass. Hence range and maximum height are equal for all bodies that are thrown with the same velocity and direction. The horizontal range d of the projectile is the horizontal distance it has traveled when it returns to its initial height ({\displaystyle y=0}).

{\displaystyle 0=v_{0}t_{d}\sin(\theta )-{\frac {1}{2}}gt_{d}^{2}}.

Time to reach ground:

{\displaystyle t_{d}={\frac {2v_{0}\sin(\theta )}{g}}}.

From the horizontal displacement the maximum distance of projectile:

{\displaystyle d=v_{0}t_{d}\cos(\theta )},

so

{\displaystyle d={\frac {v_{0}^{2}}{g}}\sin(2\theta )}.

Note that d has its maximum value when

{\displaystyle \sin 2\theta =1},

which necessarily corresponds to

{\displaystyle 2\theta =90^{\circ }},

or

{\displaystyle \theta =45^{\circ }}.

The total horizontal distance (d) traveled.

{\displaystyle d={\frac {v\cos \theta }{g}}\left(v\sin \theta +{\sqrt {(v\sin \theta )^{2}+2gy_{0}}}\right)}

When the surface is flat (initial height of the object is zero), the distance traveled:

{\displaystyle d={\frac {v^{2}\sin(2\theta )}{g}}}

Thus the maximum distance is obtained if θ is 45 degrees. This distance is:

{\displaystyle d={\frac {v^{2}}{g}}}

Application of the work energy theorem

According to the work-energy theorem the vertical component of velocity is:

{\displaystyle v_{y}^{2}=(v_{0}\sin \theta )^{2}-2gy}.

These formulae ignore aerodynamic drag and also assume that the landing area is at uniform height 0.

Angle of reach

The “angle of reach” is the angle (θ) at which a projectile must be launched in order to go a distance d, given the initial velocity v.

{\displaystyle \sin(2\theta )={\frac {gd}{v^{2}}}}

There are two solutions:

{\displaystyle \theta ={\frac {1}{2}}\arcsin \left({\frac {gd}{v^{2}}}\right)}

and

{\displaystyle \theta =90^{\circ }-{\frac {1}{2}}\arcsin \left({\frac {gd}{v^{2}}}\right)}

Angle {\displaystyle \theta } required to hit coordinate (x,y)

This section does not cite any sources. Please help improve this section by adding citations to reliable sources. Unsourced material may be challenged and removed. (October 2017) (Learn how and when to remove this template message)

To hit a target at range x and altitude y when fired from (0,0) and with initial speed v the required angle(s) of launch θ are:

{\displaystyle \theta =\arctan {\left({\frac {v^{2}\pm {\sqrt {v^{4}-g(gx^{2}+2yv^{2})}}}{gx}}\right)}}

The two roots of the equation correspond to the two possible launch angles, so long as they aren’t imaginary, in which case the initial speed is not great enough to reach the point (x,y) selected. This formula allows one to find the angle of launch needed without the restriction of {\displaystyle y=0}.

One can also ask what launch angle allows the lowest possible launch velocity. This occurs when the two solutions above are equal, implying that the quantity under the square root sign is zero. This requires solving a quadratic equation for {\displaystyle v^{2}}, and we find

{\displaystyle v^{2}/g=y+{\sqrt {y^{2}+x^{2}}}.}

This gives

{\displaystyle \theta =\arctan \left(y/x+{\sqrt {y^{2}/x^{2}+1}}\right).}

If we denote than angle whose tangent is y/x by α, then

{\displaystyle \tan \theta ={\frac {\sin \alpha +1}{\cos \alpha }}}

{\displaystyle \tan(\pi /2-\theta )={\frac {\cos \alpha }{\sin \alpha +1}}}

{\displaystyle \cos ^{2}(\pi /2-\theta )={\frac {1}{2}}(\sin \alpha +1)}

{\displaystyle 2\cos ^{2}(\pi /2-\theta )-1=\cos(\pi /2-\alpha )}

This implies

{\displaystyle \pi /2-\theta ={\frac {1}{2}}(\pi /2-\alpha ).}

In other words, the launch should be at the angle halfway between the target and the zenith.

Catching balls

This section does not cite any sources. Please help improve this section by adding citations to reliable sources. Unsourced material may be challenged and removed. (October 2017) (Learn how and when to remove this template message)

If a projectile, such as a baseball or cricket ball, travels in a parabolic path, with negligible air resistance, and if a player is positioned so as to catch it as it descends, he sees its angle of elevation increasing continuously throughout its flight. The tangent of the angle of elevation is proportional to the time since the ball was sent into the air, usually by being struck with a bat. Even when the ball is really descending, near the end of its flight, its angle of elevation seen by the player continues to increase. The player therefore sees it in line with a point ascending vertically from the batsman at constant speed. Finding the place from which the ball appears to rise steadily helps the player to position himself correctly to make the catch. If he is too close to the batsman who has hit the ball, it will appear to rise at an accelerating rate. If he is too far from the batsman, it will appear to slow rapidly, and then to descend.

Proof

Suppose the ball starts with a vertical component of velocity of v_y upward, and a horizontal component of velocity of v_x toward the player who wants to catch it. Its altitude above the ground is given by:

{\displaystyle h=v_{y}t-{\frac {1}{2}}gt^{2},} where t is the time since the ball was hit.

The total time for the flight, until the ball is back down to the ground, {\displaystyle h=0}, is given by:

{\displaystyle \therefore T={\frac {2v_{y}}{g}}.}

The horizontal component of the distance the ball travels from its starting point to time t {\displaystyle (0\leq t\leq T)} is

{\displaystyle d=v_{x}t}

The total horizontal distance the ball travels from its starting point to the point where it is caught is:

{\displaystyle D=d(T)={\frac {2v_{x}v_{y}}{g}}}

The horizontal component of the ball’s distance from the catcher at time t is:

{\displaystyle c=D-d={\frac {2v_{x}v_{y}}{g}}-v_{x}t}

The tangent of the angle of elevation of the ball, as seen by the catcher, is:

{\displaystyle \tan(e)={\frac {h}{c}}}

{\displaystyle ={\frac {v_{y}t-{\frac {1}{2}}gt^{2},}{{\frac {2v_{x}v_{y}}{g}}-v_{x}t}}}

{\displaystyle ={\frac {gt}{2v_{x}}}}

While the ball is in flight:

{\displaystyle \tan(e)=\left({\frac {g}{2v_{x}}}\right)t}

The bracket in this last expression is constant for a given flight. Therefore, the tangent of the angle of elevation of the ball, as seen by the player who is properly positioned to catch it, is directly proportional to the time since the ball was hit.

Trajectory of a projectile with air resistance

This article needs attention from an expert in Physics. Please add a reason or a talk parameter to this template to explain the issue with the article. WikiProject Physics may be able to help recruit an expert. (June 2008)

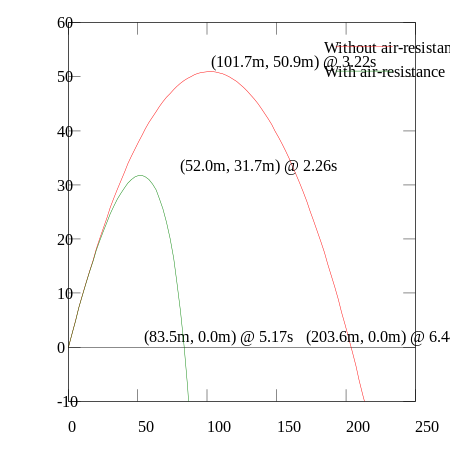
In this section we will take air resistance to be in direct proportion to the velocity of the particle (i.e. {\displaystyle F_{a}\propto {\vec {v}}}). This is only valid at Reynolds number below about 1000. In air, which has a kinematic viscosity around 0.15 cm²/s this means that the product of speed and diameter must be less than about 150 cm²/s which is obviously not usually the case. We do this though so that the equations describing the particle’s motion are easily solved. At higher values of speed times diameter (high Reynolds number) the force of air resistance is proportional to the square of the particle’s velocity (see drag equation). Here, {\displaystyle v_{0}},{\displaystyle v_{x}} and {\displaystyle v_{y}} will be used to denote the initial velocity, the velocity along the direction of x and the velocity along the direction of y, respectively. The mass of the projectile will be denoted by m. For the derivation only the case where {\displaystyle 0^{o}\leq \theta \leq 180^{o}} is considered. Again, the projectile is fired from the origin (0,0).

The free body diagram on the right is for a projectile that experiences air resistance and the effects of gravity. Here, air resistance is assumed to be in the direction opposite of the projectile’s velocity. {\displaystyle F_{air}=-kv} (actually {\displaystyle F_{air}=-kv^{2}} is more realistic, but not used here, to ensure an analytic solution,) is written due to the initial assumption of direct proportionality implies that the air resistance and the velocity differ only by a constant arbitrary factor with units of N*s/m.

The relationships that represent the motion of the particle are derived by Newton’s Second Law, both in the x and y directions. In the x direction {\displaystyle \Sigma F=-kv_{x}=ma_{x}} and in the y direction {\displaystyle \Sigma F=-kv_{y}-mg=ma_{y}}.

This implies that:

{\displaystyle a_{x}={\frac {-kv_{x}}{m}}={\frac {dv_{x}}{dt}}} (1),

and

{\displaystyle a_{y}={\frac {1}{m}}(-kv_{y}-mg)={\frac {-kv_{y}}{m}}-g={\frac {dv_{y}}{dt}}} (2)

Solving (1) is an elementary differential equation, thus the steps leading to a unique solution for v_x and, subsequently, x will not be enumerated. Given the initial conditions {\displaystyle v_{x}=v_{xo}} (where v_xo is understood to be the x component of the initial velocity) and {\displaystyle s_{x}=0} for {\displaystyle t=0}:

{\displaystyle v_{x}=v_{xo}e^{-{\frac {k}{m}}t}} (1a)

{\displaystyle s_{x}={\frac {m}{k}}v_{xo}(1-e^{-{\frac {k}{m}}t})} (1b)

While (1) is solved much in the same way, (2) is of distinct interest because of its non-homogeneous nature. Hence, we will be extensively solving (2). Note that in this case the initial conditions are used {\displaystyle v_{y}=v_{yo}} and {\displaystyle s_{y}=0} when {\displaystyle t=0}.

{\displaystyle {\frac {dv_{y}}{dt}}={\frac {-k}{m}}v_{y}-g} (2)

{\displaystyle {\frac {dv_{y}}{dt}}+{\frac {k}{m}}v_{y}=-g} (2a)

This first order, linear, non-homogeneous differential equation may be solved a number of ways; however, in this instance, it will be quicker to approach the solution via an integrating factor {\displaystyle e^{\int {\frac {k}{m}}\,dt}}.

{\displaystyle e^{{\frac {k}{m}}t}({\frac {dv_{y}}{dt}}+{\frac {k}{m}}v_{y})=e^{{\frac {k}{m}}t}(-g)} (2c)

{\displaystyle (e^{{\frac {k}{m}}t}v_{y})^{\prime }=e^{{\frac {k}{m}}t}(-g)} (2d)

{\displaystyle \int {(e^{{\frac {k}{m}}t}v_{y})^{\prime }\,dt}=e^{{\frac {k}{m}}t}v_{y}=\int {e^{{\frac {k}{m}}t}(-g)\,dt}} (2e)

{\displaystyle e^{{\frac {k}{m}}t}v_{y}={\frac {m}{k}}e^{{\frac {k}{m}}t}(-g)+C}(2f)

{\displaystyle v_{y}={\frac {-mg}{k}}+Ce^{{\frac {-k}{m}}t}} (2g)

And by integration we find:

{\displaystyle s_{y}=-{\frac {mg}{k}}t-{\frac {m}{k}}(v_{yo}+{\frac {mg}{k}})e^{-{\frac {k}{m}}t}+C} (3)

Solving for our initial conditions:

{\displaystyle v_{y}(t)=-{\frac {mg}{k}}+(v_{yo}+{\frac {mg}{k}})e^{-{\frac {k}{m}}t}} (2h)

{\displaystyle s_{y}(t)=-{\frac {mg}{k}}t-{\frac {m}{k}}(v_{yo}+{\frac {mg}{k}})e^{-{\frac {k}{m}}t}+{\frac {m}{k}}(v_{yo}+{\frac {mg}{k}})} (3a)

With a bit of algebra to simplify (3a):

{\displaystyle s_{y}(t)=-{\frac {mg}{k}}t+{\frac {m}{k}}(v_{yo}+{\frac {mg}{k}})(1-e^{-{\frac {k}{m}}t})} (3b)

An example is given using values for the mass and terminal velocity for a baseball taken from

m = 0.145 kg (5.1 oz)

v₀ = 44.7 m/s (100 mph)

g = -9.81 m/s² (-32.2 ft/s²)

v_t = -33.0 m/s (-73.8 mph)

{\displaystyle k={\frac {mg}{v_{t}}}={\frac {(0.145{\mbox{ kg}})(-9.81\ \mathrm {m} /\mathrm {s} ^{2})}{-33.0\ \mathrm {m} /\mathrm {s} }}=0.0431{\mbox{ kg}}/{\mbox{s}},\ \theta =45^{o}}.

The more realistic trajectory {\displaystyle F_{air}=-k\left\vert v\right\vert v} can not be calculated analytically, but only by numerical simulations.

Similarly to above:

{\displaystyle a_{x}={\frac {-k{v_{x}}^{2}}{m}}={\frac {dv_{x}}{dt}}}

{\displaystyle v_{x}={\begin{cases}{\frac {1}{{\frac {1}{v_{x_{o}}}}+{\frac {kt}{m}}}},&{\text{if }}v_{x_{o}}{\text{ is not 0}}\\0,&{\text{if }}v_{x_{o}}{\text{ is 0}}\end{cases}}={\frac {ds_{x}}{dt}}}

{\displaystyle s_{x}={\frac {m\ln {\left(1+{\frac {v_{x_{o}}kt}{m}}\right)}}{k}}}

However, this takes advantage of the fact that horizontally, acceleration is always negative. As acceleration is negative while velocity is positive and positive while velocity is negative, a projectile fired upwards requires the absolute value to be taken of the vertical velocity, which makes an analytical solution for vertical position more complex.

Where {\displaystyle g_{0}} is gravitational acceleration set to some constant, such as standard gravity:

{\displaystyle a_{y}={\frac {-k\left\vert v_{y}\right\vert v_{y}}{m}}-g_{0}={\frac {dv_{y}}{dt}}} for constant gravity

or, even more complex,

{\displaystyle a_{y}={\frac {-k\left\vert v_{y}\right\vert v_{y}}{m}}-g_{0}\left({\frac {r}{r+s_{y}}}\right)^{2}={\frac {dv_{y}}{dt}}} for gravity as a function of height above a planet’s surface, where

• {\displaystyle g_{0}} is the planet’s gravity at the surface.
• r is the planet’s radius.

Lofted trajectory

A special case of a ballistic trajectory for a rocket is a lofted trajectory, a trajectory with an apogee greater than the minimum-energy trajectory to the same range. In other words, the rocket travels higher and by doing so it uses more energy to get to the same landing point. This may be done for various reasons such as increasing distance to the horizon to give greater viewing/communication range or for changing the angle with which a missile will impact on landing. Lofted trajectories are sometimes used in both missile rocketry and in spaceflight